Difference between revisions of "Chapter 25 Problem 30"
From 105/106 Lecture Notes by OBM
| Line 20: | Line 20: | ||
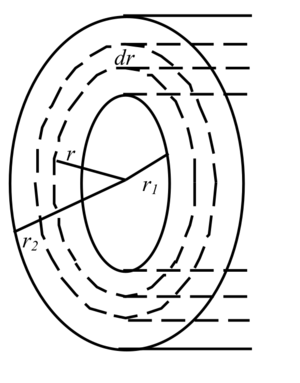
<math>R=\int dR=\int_{r_1}^{r_2} \rho \frac{dr}{2\pi r l}=\frac{\rho}{2\pi l}\ln\frac{r_2}{r_1}</math> | <math>R=\int dR=\int_{r_1}^{r_2} \rho \frac{dr}{2\pi r l}=\frac{\rho}{2\pi l}\ln\frac{r_2}{r_1}</math> | ||
| + | ===(b)=== | ||
| + | <math>R=\frac{(15 \times 10^{-5}\Omega\cdot \textrm{m}}{2\pi(0.024 \textrm{m})}\ln\left(\frac{1.8\textrm{mm}}{1.0\textrm{mm}}\right)=5.8\times10^{-4}\Omega</math> | ||
| − | ===( | + | ===(c)=== |
| − | <math>R=\frac{(15 \times 10^{-5}\Omega\cdot \textrm{m}(0.024 \textrm{m}))}{\pi\left[(1.8\times10^{-3}\textrm{m})^2-(1.0\times10^{-3}\textrm{m})^2\right]}=0.51\Omega</math> | + | <math>R=\frac{\rho l}{A}=\frac{\rho l}{\pi (r_2^2-r_1^2)}=\frac{(15 \times 10^{-5}\Omega\cdot \textrm{m}(0.024 \textrm{m}))}{\pi\left[(1.8\times10^{-3}\textrm{m})^2-(1.0\times10^{-3}\textrm{m})^2\right]}=0.51\Omega</math> |
Revision as of 03:59, 25 March 2019
Problem
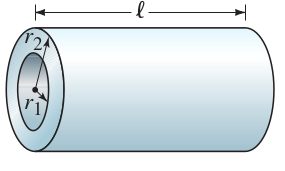
A hollow cylindrical resistor with inner radius and outer radius , and length , is made of a material whose resistivity is
(a) Show that the resistance is given by
for current that flows radially outward. [Hint: Divide the resistor into concentric cylindrical shells and integrate.]
(b) Evaluate the resistance for such a resistor made of carbon whose inner and outer radii are 1.0 mm and 1.8 mm and whose length is 2.4 cm (Choose .)
(c) What is the resistance in part (b) for current flowing parallel to the axis?
Solution
(a)
(b)
(c)










![{\displaystyle R={\frac {\rho l}{A}}={\frac {\rho l}{\pi (r_{2}^{2}-r_{1}^{2})}}={\frac {(15\times 10^{-5}\Omega \cdot {\textrm {m}}(0.024{\textrm {m}}))}{\pi \left[(1.8\times 10^{-3}{\textrm {m}})^{2}-(1.0\times 10^{-3}{\textrm {m}})^{2}\right]}}=0.51\Omega }](https://wikimedia.org/api/rest_v1/media/math/render/svg/e2519109cacd0b0c3278da1f33ceca2b5f97899b)