Difference between revisions of "106MT2Pr-solutions"
From 105/106 Lecture Notes by OBM
(Created page with "==Question 2== (a) The normal component of <math>\vec{B}</math> must be zero. (b) To find the field outside the superconductor, we place an "image dipole" inside the superc...") |
|||
| Line 1: | Line 1: | ||
| + | ==Question 1== | ||
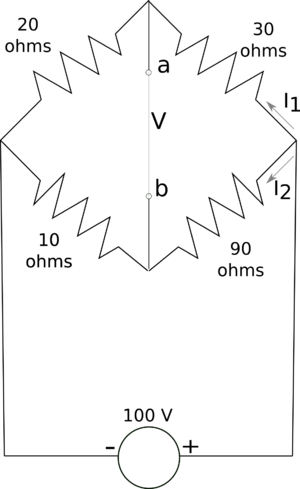
| + | [[File:106MT2-bridge-v2s1.png|thumb|center|The circuit with the capacitor removed]] | ||
| + | |||
| + | The first step in the Thévenin equivalent circuit approach is to remove the capacitor, and calculate the open circuit voltage between a and b. | ||
| + | |||
| + | <math>I_1=\frac{100 \textrm{ V}}{20+30 \textrm{ \Omega}}=2 \textrm{ A}</math> <math>I_2=\frac{100 \textrm{ V}}{10+90 \textrm{ \Omega}}=1 \textrm{ A}</math> | ||
| + | |||
| + | <math>V=20I_1-10I_2=(20 \textrm{ \Omega}) (2 \textrm{ A})-(10 \textrm{ \Omega}) (1 \textrm{ A})=30 V</math> | ||
| + | |||
| + | |||
==Question 2== | ==Question 2== | ||
Revision as of 17:00, 24 April 2019
Question 1
The first step in the Thévenin equivalent circuit approach is to remove the capacitor, and calculate the open circuit voltage between a and b.
Question 2
(a) The normal component of must be zero.
(b) To find the field outside the superconductor, we place an "image dipole" inside the superconductor a depth below the surface, oriented at an angle () from vertical. This automatically satisfies the boundary condition. The field outside the superconductor is the field due to the original dipole and the image dipole.
(c) Taking the -axis normal to , parallel to the superconducting sheet, and into the page:
(d) Stable equilibria: , . Unstable equilibria: ,
(e) away from the superconductor.