Difference between revisions of "Chapter 29 Problem 35"
From 105/106 Lecture Notes by OBM
(Created page with "==Problem== 300px|center|Wires and currents A short section of wire, of length <math>a</math>, is moving with velocity <math>\vec{v}</math> ,...") |
m |
||
| (2 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | __NOTOC__ | ||
==Problem== | ==Problem== | ||
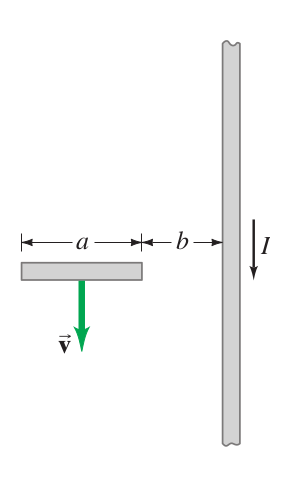
[[File:Chapter29Problem35q.png|300px|center|Wires and currents]] | [[File:Chapter29Problem35q.png|300px|center|Wires and currents]] | ||
| − | A short section of wire, of length <math>a</math>, is moving with velocity <math>\vec{v}</math> , parallel to a very long wire carrying a current <math>I</math>. The near end of the wire section is a distance <math>b</math> from the long wire. Assuming the vertical wire is very long compared to <math>a + b</math>, determine the emf between the ends of the short section. Assume <math>\vec{v}<math> is | + | A short section of wire, of length <math>a</math>, is moving with velocity <math>\vec{v}</math> , parallel to a very long wire carrying a current <math>I</math>. The near end of the wire section is a distance <math>b</math> from the long wire. Assuming the vertical wire is very long compared to <math>a + b</math>, determine the emf between the ends of the short section. Assume <math>\vec{v}</math> is |
(a) in the same direction as <math>I</math>, | (a) in the same direction as <math>I</math>, | ||
(b) in the opposite direction to <math>I</math>. | (b) in the opposite direction to <math>I</math>. | ||
| + | |||
| + | ==Solution== | ||
| + | [[File:Chapter29Problem35s.png|300px|center|Wire and current induced magnetic field]] | ||
| + | |||
| + | The magnetic field is perpendicular to the rod, with the magnetic field decreasing with distance from the rod. | ||
| + | |||
| + | ===(a)=== | ||
| + | <math>d\mathcal{E}=Bvdr</math> | ||
| + | |||
| + | <math>B=\frac{\mu_0}{2\pi}\frac{I}{r}</math> (near a straight long wire) | ||
| + | |||
| + | <math>\mathcal{E}=\int d\mathcal{E}=\int_b^{b+a}\frac{\mu_0 I}{2\pi r}v dr=\frac{\mu_0 Iv}{2\pi }\ln\left(\frac{b+a}{b}\right)</math> | ||
| + | |||
| + | The force is towards the long wire | ||
| + | |||
| + | ===(b)=== | ||
| + | same magnitude, away from the long wire | ||
Latest revision as of 22:41, 10 May 2020
Problem
A short section of wire, of length , is moving with velocity , parallel to a very long wire carrying a current . The near end of the wire section is a distance from the long wire. Assuming the vertical wire is very long compared to , determine the emf between the ends of the short section. Assume is
(a) in the same direction as ,
(b) in the opposite direction to .
Solution
The magnetic field is perpendicular to the rod, with the magnetic field decreasing with distance from the rod.
(a)
(near a straight long wire)
The force is towards the long wire
(b)
same magnitude, away from the long wire