Difference between revisions of "Chapter 26 Problem 40"
(→(a)) |
(→(a)) |
||
| Line 17: | Line 17: | ||
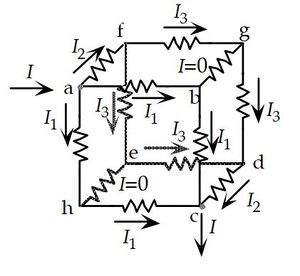
Insert a probe battery between points (a) and (b). Let's label the current drawn from this probe battery as I | Insert a probe battery between points (a) and (b). Let's label the current drawn from this probe battery as I | ||
| − | This setup has a symmetry plane between abed, so the current split at junction a will be | + | This setup has a symmetry plane between abed, so the current split at junction a will be <math>I=I_1+I_1+I_2</math> |
| − | |||
| − | <math>I=I_1+I_1+I_2</math> | ||
Another symmetry is that the afhe plane is the mirror cdgb (since one has the current I entering, and the other has the current leaving) thus we have the same currents in erverse directions in those planes. | Another symmetry is that the afhe plane is the mirror cdgb (since one has the current I entering, and the other has the current leaving) thus we have the same currents in erverse directions in those planes. | ||
| − | + | The 6 equations in the symmetry reduced cube is: | |
| − | + | #<math>I=2I_1+I_2</math> | |
| − | + | #<math>I_3+I_4=I_1</math> | |
| − | + | #<math>I_5=2I_4</math> | |
| − | + | #<math>0=-2 I_1 R -I_3 R + I_2 R</math> | |
| − | + | #<math>0=-2 I_4 R -I_5 R + I_3 R</math> | |
| + | #<math>0=\mathcal{E}-I_2 R </math> | ||
| + | |||
| + | 6 equations and 6 unknowns, so we start solving: | ||
| + | |||
| + | #<math>I=2(I_3+I_4) +\frac{\mathcal{E}}{R}</math> (2 and 6 in 1) | ||
| + | #<math>0=-2(I_3+I_4)R-I_3 R+\frac{\mathcal{E}}{R}R=-2 I_4 R-3 I_3 R+\mathcal{E}</math> (2 and 6 in 4) | ||
| + | #<math>0=-2 I_4 R-2 I_4 R+I_3 R=- 4 I_4 R + I_3 R</math> (3 in 5) | ||
| + | |||
| + | |||
| + | <math>I_3=4 I_4</math> | ||
| + | ;<math>0=-2 I_4 R - 3 (4 I_4) R+\mathcal{E} \rightarrow I_4=\frac{\mathcal{E}}{14R}</math> | ||
| + | |||
| + | <math>I=2 (4 I_4)+ 2(I_4)+\frac{\mathcal{E}}{R}=10 I_4 + \frac{\mathcal{E}}{R}=\frac{10\mathcal{E}}{14R}+\frac{\mathcal{E}}{R}</math> | ||
| + | |||
| + | <math>R_{\textrm{eq}}=\frac{\mathcal{E}}{I}=\frac{7}{12}R</math> | ||
===(b)=== | ===(b)=== | ||
Revision as of 20:25, 30 March 2019
Problem
Twelve resistors, each of resistance R, are connected as the edges of a cube. Determine the equivalent resistance
(a) between points a and b, the ends of a side;
(b) between points a and c, the ends of a face diagonal;
(c) between points a and d, the ends of the volume diagonal.
[Hint: Apply an emf and determine currents; use symmetry at junctions.]
solution
(a)
Insert a probe battery between points (a) and (b). Let's label the current drawn from this probe battery as I
This setup has a symmetry plane between abed, so the current split at junction a will be
Another symmetry is that the afhe plane is the mirror cdgb (since one has the current I entering, and the other has the current leaving) thus we have the same currents in erverse directions in those planes.
The 6 equations in the symmetry reduced cube is:
6 equations and 6 unknowns, so we start solving:
- (2 and 6 in 1)
- (2 and 6 in 4)
- (3 in 5)
(b)
Insert a probe battery between points (a) and (c). Let's label the current drawn from this probe battery as I
(c)
Insert a probe battery between points (a) and (d). Let's label the current drawn from this probe battery as I