Difference between revisions of "Chapter 28 Problem 57"
From 105/106 Lecture Notes by OBM
| Line 10: | Line 10: | ||
The magnetic field from two wires placed symmetrically on either side of where we are measuring the magnetic field has its vertical component cancelled out. | The magnetic field from two wires placed symmetrically on either side of where we are measuring the magnetic field has its vertical component cancelled out. | ||
| − | Therefore, the field above the wire must be horizontal and to the left. | + | Therefore, the field above the wire must be horizontal and to the left with magnitude <math>B_\parallel</math>. |
By symmetry, the field a location y below the wire must have the same magnitude, but point in the opposite direction. | By symmetry, the field a location y below the wire must have the same magnitude, but point in the opposite direction. | ||
Latest revision as of 07:53, 16 April 2019
Problem
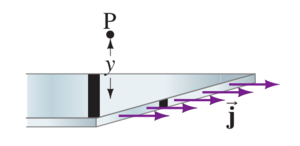
A very large flat conducting sheet of thickness carries a uniform current density throughout. Determine the magnetic field (magnitude and direction) at a distance above the plane. (Assume the plane is infinitely long and wide.)
Solution
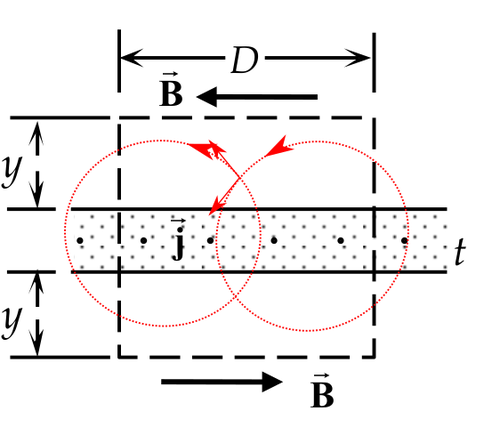
The sheet may be treated as an infinite number of parallel wires.
The magnetic field from two wires placed symmetrically on either side of where we are measuring the magnetic field has its vertical component cancelled out.
Therefore, the field above the wire must be horizontal and to the left with magnitude .
By symmetry, the field a location y below the wire must have the same magnitude, but point in the opposite direction.