Difference between revisions of "Chapter 30 Problem 13"
From 105/106 Lecture Notes by OBM
| Line 15: | Line 15: | ||
<math>\oint \vec{B}.d\vec{l}=\mu_0 I_{\textrm{encl}}</math> | <math>\oint \vec{B}.d\vec{l}=\mu_0 I_{\textrm{encl}}</math> | ||
| − | <math>B (2 \pi r)=\mu_0 NI | + | <math>B (2 \pi r)=\mu_0 NI</math> |
<math>B=\frac{\mu_0 NI}{(2 \pi r)}</math> | <math>B=\frac{\mu_0 NI}{(2 \pi r)}</math> | ||
Latest revision as of 21:49, 5 May 2019
Problem
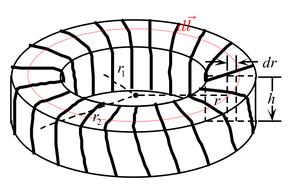
A toroid has a rectangular cross section. Show that the self-inductance is
where is the total number of turns and , , and are shown above. [Hint: Use Ampère’s law to get as a function of inside the toroid, and integrate.]
Solution
Magnetic field inside as a function of :
the self-inductance is related to the flux is the integral of the magnetic field over a cross-section of the toroid.