(diff) ← Older revision | Latest revision (diff) | Newer revision → (diff)
Problem
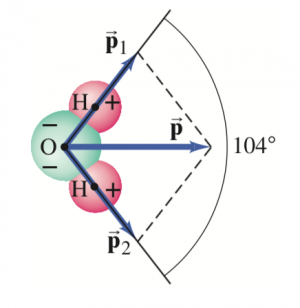
The dipole moment, considered as a vector, points from the negative to the positive charge. The water molecule has a dipole moment  which is due to two dipole moments
which is due to two dipole moments  and
and  as shown. The distance between each H and the O is about
as shown. The distance between each H and the O is about  m the lines joining the center of the O atom with each H atom make an angle of 104° as shown, and the net dipole moment has been measured to be
m the lines joining the center of the O atom with each H atom make an angle of 104° as shown, and the net dipole moment has been measured to be  .
.
(a) Determine the effective charge q on each H atom.
(b) Determine the electric potential, far from the molecule, due to each dipole,  and
and  , and show that
, and show that

where  is the magnitude of the net dipole moment,
is the magnitude of the net dipole moment,  ,and V is the total potential due to both
,and V is the total potential due to both  and
and  .Take
.Take  at
at  .
.
Solution

around the H20 in polar coordinates
(a)
Since the interatomic distance and the charge distrubution is the same, 



(b)
using the polar coordinates, any point of interest can be represented as  . Since we know the angle between
. Since we know the angle between  and
and  , it is easy to relate the point of interest to the dipole moments. Since the potential is a scalar quantity, the potential at point of interest is simply the scalar summation of the potential due to
, it is easy to relate the point of interest to the dipole moments. Since the potential is a scalar quantity, the potential at point of interest is simply the scalar summation of the potential due to  and
and 


![{\displaystyle ={\frac {1}{4\pi \epsilon _{0}r}}{\frac {p}{2\cos(52^{\circ })}}\left[\cos(52^{\circ }-\theta )+\cos(52^{\circ }+\theta )\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/01e219c0ec2c927c95176671c363998cac0158ff)
![{\displaystyle ={\frac {1}{4\pi \epsilon _{0}r}}{\frac {p}{2\cos(52^{\circ })}}\left[\cos(52^{\circ })\cos(\theta )+\sin(52^{\circ })\sin(\theta )+\cos(52^{\circ })\cos(\theta )-\sin(52^{\circ })\sin(\theta )\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3515b7a303da71974140735487161f6826e614bd)



















![{\displaystyle ={\frac {1}{4\pi \epsilon _{0}r}}{\frac {p}{2\cos(52^{\circ })}}\left[\cos(52^{\circ }-\theta )+\cos(52^{\circ }+\theta )\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/01e219c0ec2c927c95176671c363998cac0158ff)
![{\displaystyle ={\frac {1}{4\pi \epsilon _{0}r}}{\frac {p}{2\cos(52^{\circ })}}\left[\cos(52^{\circ })\cos(\theta )+\sin(52^{\circ })\sin(\theta )+\cos(52^{\circ })\cos(\theta )-\sin(52^{\circ })\sin(\theta )\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3515b7a303da71974140735487161f6826e614bd)
