Chapter 22 Problem 30

Problem

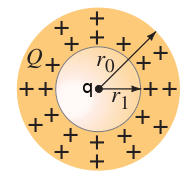
Non-conducting sphere with a charge inside

A nonconducting sphere has a spherical cavity of radius $r_{1}$ centered at the sphere’s center. Assuming the charge $Q$ is distributed uniformly in the “shell” (between $r=r_{1}$ and $r=r_{0}$ ), determine the electric field as a function of $r$ for

(a) $0<r<r_{1}$

(b) $r_{1}<r<r_{0}$

(c) $r>r_{0}$

Solution

Superposition principle

${\vec {E}}_{T}={\vec {E}}_{\textrm {sphere}}+{\vec {E}}_{q}$

$E_{q}={\frac {q}{4\pi \epsilon _{0}r^{2}}}$ (radially pointing from the charge)

$E_{\textrm {sphere}}\rightarrow \oint {\vec {E}}\cdot d{\vec {A}}=E(4\pi r^{2})={\frac {Q_{\textrm {encl}}}{\epsilon _{0}}}$ (radial direction)

$E_{\textrm {sphere}}={\frac {Q_{\textrm {encl}}}{4\pi \epsilon _{0}r^{2}}}$

so the question is about finding the $Q_{\textrm {encl}}$

(a)

$E_{\textrm {sphere}}=0$

${\vec {E}}_{T}={\vec {E}}_{q}={\frac {q}{4\pi \epsilon _{0}r^{2}}}{\hat {r}}$

(b)

First, let's calculate the uniform charge density $\rho$ in terms of the charge held by the shell $Q_{\textrm {shell}}$.

$Q_{\textrm {shell}}=\rho \left({\frac {4}{3}}\pi r_{0}^{3}-{\frac {4}{3}}\pi r_{1}^{3}\right)$

$\rho ={\frac {3Q_{\textrm {shell}}}{4\pi (r_{0}^{3}-r_{1}^{3})}}$

using this, we can calculate $Q_{\textrm {encl}}$

$E_{\textrm {sphere}}={\frac {Q_{\textrm {encl}}}{4\pi \epsilon _{0}r^{2}}}={\frac {\rho \left[{\frac {4}{3}}\pi r^{3}-{\frac {4}{3}}\pi r_{1}^{3}\right]}{4\pi \epsilon _{0}r^{2}}}$

$={\frac {Q_{\textrm {sphere}}}{4\pi \epsilon _{0}r^{2}}}{\frac {r^{3}-r_{1}^{3}}{r_{0}^{3}-r_{1}^{3}}}$

${\vec {E}}_{T}={\vec {E}}_{\textrm {sphere}}+{\vec {E}}_{q}={\frac {1}{4\pi \epsilon _{0}r^{2}}}\left[q+Q_{\textrm {sphere}}{\frac {r^{3}-r_{1}^{3}}{r_{0}^{3}-r_{1}^{3}}}\right]{\hat {r}}$

(c)

This is straightforward using Gauss's law and a surface that encompasses all the charges outside the shell

${\vec {E}}_{T}={\vec {E}}_{\textrm {sphere}}+{\vec {E}}_{q}={\frac {Q_{\textrm {encl}}}{4\pi \epsilon _{0}r^{2}}}+{\frac {q}{4\pi \epsilon _{0}r^{2}}}$