Difference between revisions of "Chapter 29 Problem 73"
From 105/106 Lecture Notes by OBM
| Line 4: | Line 4: | ||
==Solution== | ==Solution== | ||
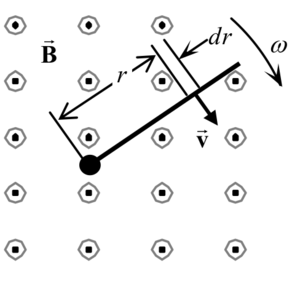
| − | [[File:Chapter29Problem73s.png| | + | [[File:Chapter29Problem73s.png|300px|center|Rotation and flux]] |
<math>d\mathcal{E}=Bvdr=B\omega r dr </math> | <math>d\mathcal{E}=Bvdr=B\omega r dr </math> | ||
<math>\mathcal{E}=\int d\mathcal{E}=\int_0^{l}B\omega r dr=\frac{1}{2}B\omega l^2</math> | <math>\mathcal{E}=\int d\mathcal{E}=\int_0^{l}B\omega r dr=\frac{1}{2}B\omega l^2</math> | ||
Latest revision as of 07:52, 30 April 2019
Problem
A thin metal rod of length rotates with angular velocity about an axis through one end. The rotation axis is perpendicular to the rod and is parallel to a uniform magnetic field . Determine the emf developed between the ends of the rod
Solution
